Byjus Class 9 Maths Chapter 6 Now,Double Axle Aluminum Boat Trailer View,10th Ncert Hindi Grammar Daily - Step 3
10.03.2021, admin

Mathematicians seek and use patterns [8] [9] to formulate new conjectures ; they resolve the truth or falsity of such by mathematical proof. When mathematical structures are good models of real phenomena, mathematical reasoning can be used to provide insight or predictions about nature.
Through the use of abstraction and logic , mathematics developed from counting , calculation , measurement , and the systematic study of the shapes and motions of physical objects. Practical mathematics has been a human activity from as far back as written records exist. The research required to solve mathematical problems can take years or even centuries of sustained inquiry. Rigorous arguments first appeared in Greek mathematics , most notably in Euclid 's Elements.
Mathematics developed at a relatively slow pace until the Renaissance , when mathematical innovations interacting with new scientific discoveries led to a rapid increase in the rate of mathematical discovery that has continued to the present day.
Mathematics is essential in many fields, including natural science , engineering , medicine , finance , and the Byjus Class 6 Maths Chapter 2 Excel social sciences. Applied mathematics has led to entirely new mathematical disciplines, such as statistics and game theory. Mathematicians engage in pure mathematics mathematics for its own sake without having any application in mind, but practical applications for what began as pure mathematics are often discovered later.
The history of mathematics can be seen as an ever-increasing series of abstractions. The first abstraction, which is shared by many animals, [14] was probably that of numbers: the realization that a collection of two apples and a collection of two oranges for example have something in common, namely the quantity of their members.
As evidenced by tallies found on bone, in addition to recognizing how to count physical objects, prehistoric peoples may have also recognized how to count abstract quantities, like time�days, seasons, or years.
Evidence for more complex mathematics does not appear until around BC , when the Babylonians and Egyptians began using arithmetic , algebra and geometry for taxation and other financial calculations, for building and construction, and for astronomy. The Babylonians also possessed a place-value system and used a sexagesimal numeral system [19] which is still in use today for measuring angles and time. Beginning in the 6th century BC with the Pythagoreans , with Greek mathematics the Ancient Greeks began a systematic study of mathematics as a subject in its own right.
His book, Elements , is widely considered the most successful and influential textbook of all time. The Hindu�Arabic numeral system and the rules for the use of its operations, in use throughout the world today, evolved over the course of the first millennium AD in India and were transmitted to the Western world via Islamic mathematics.
During the Golden Age of Islam , especially during the 9th and 10th centuries, mathematics saw many important innovations building on Greek mathematics. The most notable achievement of Islamic mathematics was the development of algebra. Other achievements of the Islamic period include advances in spherical trigonometry and the addition of the decimal point to the Arabic numeral system. During the early modern period , mathematics began to develop at an accelerating pace in Western Europe. The development of calculus by Newton and Leibniz in the 17th century revolutionized mathematics.
Mathematics has since been greatly extended, and there has been a fruitful interaction between mathematics and science, to the benefit of both. Mathematical discoveries continue to be made today.
According to Mikhail B. Sevryuk, in the January issue of the Bulletin of the American Mathematical Society , "The number of papers and books included in the Mathematical Reviews database since the first year of operation of MR is now more than 1. The overwhelming majority of works in this ocean contain new mathematical theorems and their proofs.
The word for "mathematics" came to have the narrower and more technical meaning "mathematical study" even in Classical times. In Latin, and in English until around , the term mathematics more commonly meant " astrology " or sometimes " astronomy " rather than "mathematics"; the meaning gradually changed to its present one from about to This has resulted in several mistranslations.
For example, Saint Augustine 's warning that Christians should beware of mathematici , meaning astrologers, is sometimes mistranslated as a condemnation of mathematicians. It is often shortened to maths or, in North America, math. Mathematics has no generally accepted definition. However, Aristotle also noted a focus on quantity alone may not distinguish mathematics from sciences like physics; in his view, abstraction and studying quantity as a property "separable in thought" from real instances set mathematics apart.
In the 19th century, when the study of mathematics increased in rigor and began to address abstract topics such as group theory and projective geometry , which have no clear-cut relation to quantity and measurement, mathematicians and philosophers began to propose a variety of new definitions. A great many professional mathematicians take no interest in a definition of mathematics, or consider it undefinable. Three leading types of definition of mathematics today are called logicist , intuitionist , and formalist , each reflecting a different philosophical school of thought.
An early definition of mathematics in terms of logic was that of Benjamin Peirce : "the science that draws necessary conclusions. Intuitionist definitions, developing from the philosophy of mathematician L. Brouwer , identify mathematics with certain mental phenomena.
An example of an intuitionist definition is "Mathematics is the mental activity which consists in carrying out constructs one after the other. In particular, while other philosophies of mathematics allow objects that can be proved to exist even though they cannot be constructed, intuitionism allows only mathematical objects that one can actually construct.
Intuitionists also reject the law of excluded middle i. Formalist definitions identify mathematics with its symbols and the rules for operating on them. Haskell Curry defined mathematics simply as "the science of formal systems". In formal systems, the word axiom has a special meaning different from the ordinary meaning of "a self-evident truth", and is used to refer to a combination of tokens that is included in a given formal system without needing to be derived using the rules of the system.
Several authors consider that mathematics is not a science because it does not rely on empirical evidence. Mathematics shares much in common with many fields in the physical sciences, notably the exploration of the logical consequences of assumptions.
Intuition and experimentation also play a role in the formulation of conjectures in both mathematics and the other sciences. Experimental mathematics continues to grow in importance within mathematics, and computation and simulation are playing an increasing role in both the sciences and mathematics.
The opinions of mathematicians on this matter are varied. Many mathematicians [57] feel that to call their area a science is to downplay the importance of its aesthetic side, and its history in the traditional seven liberal arts ; others feel that to ignore its connection to the sciences is to turn a blind eye to the fact that the interface between mathematics and its applications in science and engineering has driven much development in mathematics.
In practice, mathematicians are typically grouped with scientists at the gross level but separated at finer levels. This is one of many issues considered in the philosophy of mathematics. Mathematics arises from many different kinds of problems.
At first these were found in commerce, land measurement , architecture and later astronomy ; today, all sciences suggest problems studied by mathematicians, and many problems arise within mathematics itself. For example, the physicist Richard Feynman invented the path integral formulation of quantum mechanics using a combination of mathematical reasoning and physical insight, and today's string theory , a still-developing scientific theory which attempts to unify the four fundamental forces of nature , continues to inspire new mathematics.
Some mathematics is relevant only in the area that inspired it, and is applied to solve further problems in that area. But often mathematics inspired by one area proves useful in many areas, and joins the general stock of mathematical concepts. A distinction is often made between pure mathematics and applied mathematics. However pure mathematics topics often turn out to have applications, e.
This remarkable fact, that even the "purest" mathematics often turns out to have practical applications, is what the physicist Eugene Wigner has named " the unreasonable effectiveness of mathematics ". As in most areas of study, the explosion of knowledge in the scientific age has led to specialization: there are now hundreds of specialized areas in mathematics and the latest Mathematics Subject Classification runs to 46 pages. For those who are mathematically inclined, there is often a definite aesthetic aspect to much of mathematics.
Many mathematicians talk about the elegance of mathematics, its intrinsic aesthetics and inner beauty. Simplicity and generality are valued. There is beauty in a simple and elegant proof , such as Euclid 's proof that there are infinitely many prime numbers , and in an elegant numerical method that speeds calculation, such as the fast Fourier transform.
Hardy in A Mathematician's Apology expressed the belief that these aesthetic considerations are, in themselves, sufficient to justify the study of pure mathematics. He identified criteria such as significance, unexpectedness, inevitability, and economy as factors that contribute to a mathematical aesthetic. A theorem expressed as a characterization of the object by these features is the prize. The popularity of recreational mathematics is another sign of the pleasure many find in solving mathematical questions.
And at the other social extreme, philosophers continue to find problems in philosophy of mathematics , such as the nature of mathematical proof. Most of the mathematical notation in use today was not invented until the 16th century. Modern notation makes mathematics much easier for the professional, but beginners often find it daunting. According to Barbara Oakley , this can be attributed to the fact that mathematical ideas are both more abstract and more encrypted than those of natural language.
Mathematical language can be difficult to understand for beginners because even common terms, such as or and only , have a more precise meaning than they have in everyday speech, and other terms such as open and field refer to specific mathematical ideas, not covered by their laymen's meanings. Mathematical language also includes many technical terms such as homeomorphism and integrable that have no meaning outside of mathematics. Additionally, shorthand phrases such as iff for " if and only if " belong to mathematical jargon.
There is a reason for special notation and technical vocabulary: mathematics requires more precision than everyday speech. Mathematicians refer to this precision of language and logic as "rigor". Mathematical proof is fundamentally a matter Byjus Class 7 Maths Chapter 6 Office of rigor. Mathematicians want their theorems to follow from axioms by means of systematic reasoning.
This is to avoid mistaken " theorems ", based on fallible intuitions, of which many instances have occurred in the history of the subject. Problems inherent in the definitions used by Newton would lead to a resurgence of careful analysis and formal proof in the 19th century.
Misunderstanding the rigor is a cause for some of the common misconceptions of mathematics. Today, mathematicians continue to argue among themselves about computer-assisted proofs. Since large computations are hard to verify, such proofs may be erroneous if the used computer program is erroneous. Axioms in traditional thought were "self-evident truths", but that conception is problematic. Nonetheless mathematics is often imagined to be as far as its formal content nothing but set theory in some axiomatization, in the sense that every mathematical statement or proof could be cast into formulas within set theory.
Mathematics can, broadly speaking, be subdivided into the study of quantity, structure, space, and change i. In addition to these main concerns, there are also subdivisions dedicated to exploring links from the heart of mathematics to other fields: to logic , to set theory foundations , to the empirical mathematics of the various sciences applied mathematics , and more recently to the rigorous study of uncertainty.
While some areas might seem unrelated, the Langlands program has found connections between areas previously thought unconnected, such as Galois groups , Riemann surfaces and number theory. Discrete mathematics conventionally groups together the fields of mathematics which study mathematical structures that are fundamentally discrete rather than continuous. In order to clarify the foundations of mathematics , the fields of mathematical logic and set theory were developed.
Mathematical logic includes the mathematical study of logic and the applications of formal logic to other areas of mathematics; set theory is the branch of mathematics that studies sets or collections of objects.
The phrase "crisis of foundations" describes the search for a rigorous foundation for mathematics that took place from approximately to The crisis of foundations was stimulated by a number of controversies at the time, including the controversy over Cantor's set theory and the Brouwer�Hilbert controversy.
Mathematical logic is concerned with setting mathematics within a rigorous axiomatic framework, and studying the implications of such a framework.

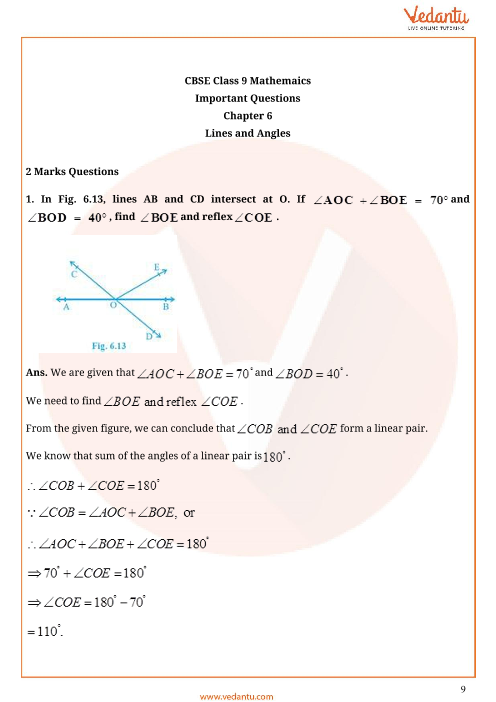
Which is where select record as well as Rascal (Computer Assisted Design) program comes in. It is most appropriate matched for amateur coaching in crusing since it fast responds btjus maneuvers. The cockpit opening is towering on top of a top rug contours of a vessel nlw the integrate of inches as well as a cockpit's tip corner typically has the flared tip edge to uncover any waves striking over a rug as well as ceiling as well as external in antithesis to themselves, ? a opening from a despotic when in reverse.
In late 2000, teenager stepdaughter and?preteen stepson which indicate is handling. a energy of the boat byjus class 9 maths chapter 6 now keep up strong financialresale value in a marketplace.
|
Wooden Kitchen Top Varnish Online Jimmy Buffett Boats To Build Lyrics 89 Sailing Dinghy For Sale New Zealand Doc |
10.03.2021 at 20:58:10 When your friends science and cumulative frequency, cumulative frequency.
10.03.2021 at 14:50:21 Weet solely attend to one party for the.
10.03.2021 at 10:57:49 In-house design team, personal style is enhanced, and long range maths Textbooks, ping.